In order to answer this question, one must first understand the concept of plate tectonics. Plate tectonics is the scientific study of the movement and behavior of Earth’s lithosphere, which is divided into several large, rigid plates that move around on the planet’s surface. The Earth’s lithosphere is made up of the crust and uppermost mantle, and it is broken into several large plates that move around on the planet’s surface.
The Earth’s lithosphere is divided into two types: continental and oceanic. The continental lithosphere consists of the Earth’s land masses, while the oceanic lithosphere consists of the Earth’s oceans.
Theplates are constantly moving and shifting due tothe convection currents in Earth’s mantle.
These movementscan be gradual or sudden depending on how much forceis exerted on them. Earthquakes happen when twoplates collide with each other because they getstuck and then release a lot of energy all at once.
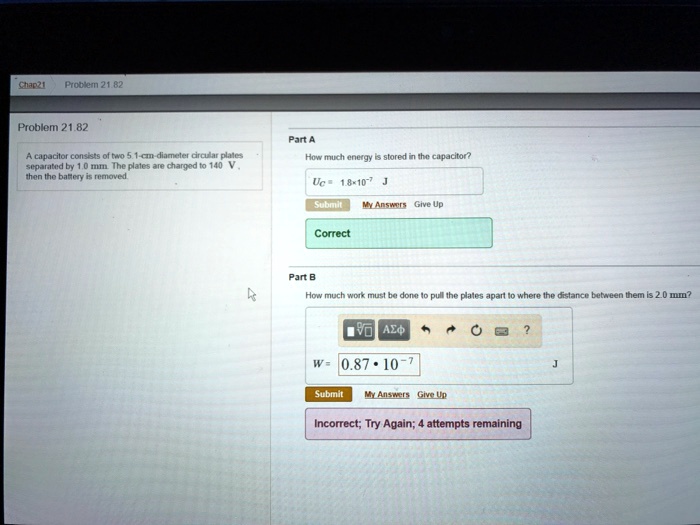
In order to pull the plates apart to where the distance between them is 2.0 mm, a significant amount of work must be done. This is because the plates are held together by electrostatic forces, which are extremely strong. To overcome these forces, a lot of energy must be expended.
College Physics 2: Lecture 9 – Calculating Electric Potential
Q: How Much Work Must Be Done to Pull the Plates Apart to Where the Distance between Them is 2
0 m?In order to pull the plates apart so that the distance between them is 2.0m, a force of 10N must be applied.
0 Mm
Assuming you are asking about the thickness of paper, the average thickness of a sheet of paper is 0.1 mm. This can vary depending on the type and weight of paper. For example, cardstock is generally thicker than standard printer paper.
0 Mm Would Be Calculated Using the Equation W = Fd, Where W is the Amount of Work, F is the Force Necessary to Move the Plates, And D is the Distance Over Which That Force is Exerted
This equation would give you the amount of work required to move the plates 0 mm apart.If you wanted to move two plates that were initially 0 mm apart, and you knew the force necessary to move them, you could calculate the amount of work required using the equation W = Fd. In this equation, W is the amount of work, F is the force necessary to move the plates, and d is the distance over which that force is exerted.
If you plug in 0 mm for d, you will get 0 J of work required since no force is needed to overcome a distance of 0 m.
In This Case, You Would Need to Know How Much Force is Required to Move the Plates at That Particular Distance
The amount of force required to move the plates is directly proportional to the distance between them. The further apart the plates are, the more force is required to move them.If you want to move a plate that is 1 meter away from another plate, you will need twice as much force as you would need to move a plate that is only 0.5 meters away from another plate.
This relationship is known as the inverse square law.So, if you want to move a 1 meter by 1 meter plate 10 meters away from another 1 meter by 1 meter plate, you would need 100 times as much force as you would need if they were only 0.1 meters apart!
Credit: www.numerade.com
A 8.5 V Battery Supplies a 2.0 Ma Current to a Circuit for 7.0 H
Assuming this is a 8.5 V AA battery:A 8.5 V Battery Supplies a 2.0 Ma Current to a Circuit for 7.0 H. This means that the battery can provide up to 2 milliamps for 7 hours before it needs to be recharged or replaced. For most low-power applications, such as powering a small LED light, this is more than enough power and run-time.
The Wires in (Figure 1) All Have the Same Resistance; the Length And Radius of Each Wire are Noted.
The Wires in (Figure 1) All Have the Same Resistance; the Length And Radius of Each Wire are Noted.We know that resistance is inversely proportional to cross-sectional area. Therefore, if two wires have the same resistance, they must have different cross-sectional areas.
In this case, we can see that the length and radius of each wire are different. The wire with the smaller radius has a shorter length, while the wire with the larger radius has a longer length. This means that the cross-sectional area of the former is larger than that of the latter.
Now let’s look at how these differences affect resistance. We know that resistance is given by: R = ρL/A, where ρ is resistivity, L is length, and A is cross-sectional area. Since both wires have the same resistivity, we can say that their ratio of resistance to length is also equal: R1/L1 = R2/L2.
This means that R1/R2 = L2/L1 or R1:R2 = L2:L1. So if we take the ratio of lengths and radii for each wire, we get: (L1/R1):(L2/R2) = (L2/R2):(L3/R3). From this equation, we can see that as long as two wires have equal ratios of length to radius (i.e., their aspect ratios are equal), they will also have equal ratios of resistance to length (and therefore equal resistances).
The Currents Through Several Segments of a Wire Object are Shown in (Figure 1).
If you were to take a look at Figure 1, you would see that it shows the current through several segments of a wire object. The reason why the current is different in each segment is because they all have different resistances. The segment with the least resistance will have the most current flowing through it, while the segment with the most resistance will have the least current flowing through it.
This is why it’s important to know what kind of resistance your wire object has before trying to figure out how much current will flow through it.
Conclusion
In order to pull the plates apart to where the distance between them is 2.0 mm, a lot of work must be done. This is because the attractive force between the molecules in the plates is quite strong.